General principles and laws in solid mechanics: Newton’s law of mechanics, the law of transmissibility, parallelogram law of addition, principle of superposition and moment of forces

By -

Since we have to study the deformation and motion of solid objects under the action of forces, we should have knowledge about the force as a vector quantity and its moment and along with this we should have perfect knowledge of different principles involved in solid mechanics.

Newtonian’s mechanics is used while solving an engineering problem so; one should have knowledge of Newton’s laws of mechanics:

It involves Newton’s three laws of motion and law of universal gravitation:

Newton’s first law of motion 

It states that any object whether is in rest or motion will remain as such until it is forced to change its state of rest or motion by an external agency.

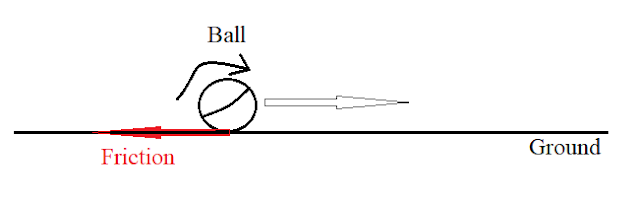
Consider a ball rolling on the ground; we all know that after a certain period of time it will come to rest but according to Newton’s first law of motion, it should have continued in motion unless some external agency is forcing it to change its state from motion to rest. And, we all know that it is friction.


If somehow one can create a surface free from friction then the ball will not come to rest and continue its motion until some other external agency forces it to stop.

Similarly, if a body is in rest then it will not move until it is applied certain force.

Newton’s second law of motion

It states that if a force is applied on an object then the amount of force will be proportional to the product of mass and rate of change of velocity i.e.

Newton’s third law of motion

It states that every action performed has an equal and opposite reaction.

Let’s understand it with a simple example,

If a person is trying to push a concrete wall then we know that it will not move a bit but according to second law if a force F is applied to a body then there must be an acceleration ‘a’ such that

                                                          

Unless there is an equal and opposite reaction that is canceling out the force applied by the person. So,

 Fnet = 0, hence a = 0

Now, coming to the universal law of gravitation

Newton’s law of universal gravitation

It states that any two body separated by a distance ‘d’ exert an attractive force on each other that is directly proportional to the product of their masses and inversely proportional to the square of distance between them i.e.

                                                           

Where, G is the universal gravitational constant which is equal to 6.67*10-11 m3kg-1s-2 .

Now, consider an object of mass ‘m’ at the surface of the earth. The gravitational force Fe on the object will be given by

                                                              

Where, Me is the mass of the earth

And, R is the radius of the earth which has been taken the distance between the earth and the object while calculating the gravitational force.

Now, if the gravitation acceleration due to Fe  is ‘g’ on the surface of the earth, then from Newton’s second law of motion

Fe = mg = weight of the object since it is on the surface of the earth

Note: Weight of an object is the force exerted by the earth on that object on the surface of the earth.

From the above two equations,

                                                          

g = 9.81

Force

Since force is a vector quantity; we will study some laws and principles of vector

Law of transmissibility

It states that if a force is applied to a rigid object then there will not be any change on  the effect of force on that object when that same amount of force is applied somewhere else on the object along the same line of action.


Line of action

It is an imaginary line that is drawn as the extension of the force vector from both ends.

In the above figure, if the force applied at point A on an object is transferred to the Point B that is along the line of action of force will have the same effect on the object.

The principal of physical independence

It states that the effect of a force on a body will remain unaffected by the other forces acting on the body. It means that each force on a body will act individually on the body and the net effect on the body will be the resultant of the effect of all the forces acting individually on the body.

Principal of superposition

It states that the net effect of forces acting on a body will be same as the sum of the effects caused by each force individually on the body.

Parallelogram law of addition of vectors

Any vector quantity along with force follows parallelogram law of addition in nature.

Let’s consider two vectorsand , the sum of the two vectors is by the parallelogram of vectors

                                                             

It states that the resultant  of two vectors  and  will be equal to the magnitude and direction of the diagonal of a parallelogram formed by the magnitude and direction of the vectors  and  as adjacent sides.


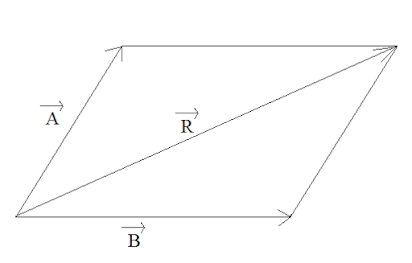Parallelogram law of addition of two vectors

Now, consider the two forces  and , then the resultant of the two will be given as

                                                           
Parallelogram law of addition of two forces


Moment of forces

Moment of force is always calculated along a center and it is defined as the product of the magnitude of the force and the perpendicular distance from a point to the line of action of that force.

Note, that the moment of force is a vector quantity and the product above will be the cross product of vector as given below

                                                               

Now, the Algebraic sum of the moment of forces about a center is always equal to the moment generated by the resultant of those forces.

To, physically understand the moment of force, consider a hinged door. Now if a force F is applied on the door at a certain perpendicular distance ‘d’ from the axis of rotation then the door might move along its axis and if the distance ‘d’ is decreased with the same amount of force then, at some point it will be hard to rotate the door. It is because as the distance from the axis decreased, the moment decreased though the force was kept constant. 

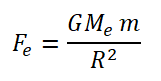
Comments

Post a Comment

Your comment will be moderated.

Popular posts from this blog

Indian Road Congress (IRC) and Bridge Loading Standards: IRC Class AA, 70R, A and B Loading

By -
Image
Indian Road Congress Indian Road Congress (IRC) is the governing body which decides the rules and regulation along with technical details regarding roads, highways, and bridges. The first loading standard in India was published by IRC in 1958 and subsequently reprinted in 1962 and 1963.   The metric version was introduced in the second revision published in 1964. IRC Bridge Loading Standards The standard IRC loads specified in IRC: 6-2000 are not changed since 1958 and grouped under four categories as detailed below: 1. IRC Class AA Loading In this category, two types of vehicles are specified and they are grouped as tracked vehicle and wheeled vehicles. The tracked vehicle simulating an army tank of 700 KN and wheeled vehicle of 400 KN (a heavy army truck) are standardized for the designing of all the bridges located on National Highways and State Highways. 2. IRC Class 70R Loading The following vehicles are considered for the design under this category: (a) ...
Read more

Slope failures, types and causes of slope failures

By -
Image
Introduction Slope failure is generally the failure of sloped surfaces due to physical factors that may result in loss of properties and even life. Why do we need to understand slope failures? You must have heard about plain and non-plain areas. So, if we talk about it the plain areas are those where the earth or lands do not show any elevation or slight elevations that can be neglected. Non-plain areas are those where the lands have sufficiently larger elevations that need to be considered for any activity. Now, if any land has elevations then it forms slope. For example, consider mountains they generally have slopes though it may be narrow or steep but if any kind of construction or any other activity, if happens there, then, these slopes need to be taken into account and how are they going to affect the outputs of the work going on. But here we will not talk about the difficulty faced for routes and inaccessibility of the area rather we will talk about the physical difficu...
Read more

Truss: Simple, Deficient and Redundant Truss & Determinate and Indeterminate Truss

By -
Image
  Truss A truss is basically a pin-jointed slender member structure that is used to carry loads. You might have noticed the use of trusses in supporting roofs and bridge decks. The roof trusses and bridge trusses generally use trusses of steel, however, wooden trusses are also used in supporting various structures that are not too heavy. Steel trusses are also used in transmission towers. But notice one thing, in most of the roof and bridge trusses, the members of the truss lies in a single plane whereas, in transmission towers, the truss members do not lie in a single plane.  So, the trusses which have all the members in a single plane are known as plane truss otherwise the truss is known as space truss. In trusses, the connection is supposed to be pin-jointed but in reality, no extra effort is made to make it so. However, the experiments have shown that the results are consistent with the results obtained by considering the joint as a pin joint. Pin-jointed connection ...
Read more